The triangle sum theorem establishes the common sum shared by the three interior triangles of any triangle. Through this theorem, it is now possible to find unknown angle measures of triangles and other polygons. Since the triangle sum theorem opens a wide range of applications and other geometric properties, it is important to learn and know this theorem by heart.
This article covers all the fundamentals needed to master this topic. Know when to use the triangle sum theorem and learn how to apply it to solve for unknown angle measures. This discussion is going to be fun, so when you’re ready, head over to the conditions for the triangle sum theorem!
What Is the Triangle Sum Theorem?
The triangle sum theorem states that the three interior angles of any triangle will always add up to 180o. Suppose that a triangle has the following interior angles: \angle A, \angle B, and \angle C. Through the triangle sum theorem, \angle A+ \angle B + \angle C= 180\degree.

This theorem applies to all types of triangles, so this shows how important this theorem is as it opens a wide range of properties and applications. Take a look at the two triangles shown below – \Delta ABC is a scalene triangle and \Delta MNP is a right triangle.

As we have learned, the triangle sum theorem states that the sum of the interior angles of each of these triangles will be 180o. Test this out for yourself by adding these angles and see the beauty of this theorem!
| ∆ABC | \angle A + \angle B + \angle C = 60\degree +50\degree + 70\degree =180\degree |
| ∆MNP | \angle M + \angle N + \angle P = 40\degree +90\degree + 50\degree =180\degree |
Knowing this interesting property about triangles opens a wide range of applications. Given two interior angles of a triangle, it is now possible to find the measure of the third interior angle. First, try out this problem by remembering a triangle’s interior angles can only have one sum: 180o.
Problem 1
Which of the following triangles do not have valid interior angle measures?
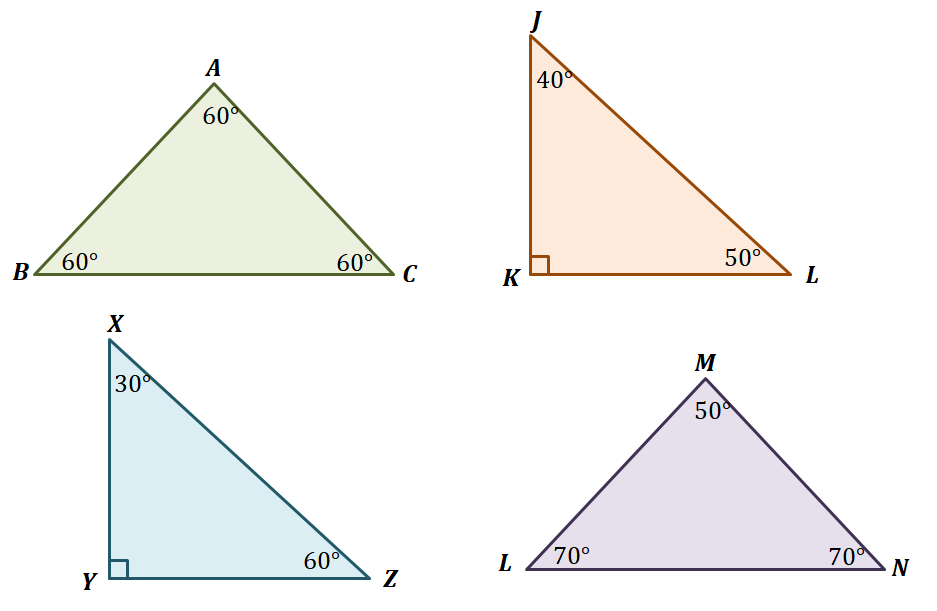
As established earlier, the sum of any triangles’ interior angles must be 180o. This means that if the interior angles do not add up to 180o, the triangle doesn’t have valid interior angle measures. Use this fact when identifying the triangle we’re looking for and begin by adding the interior angles for each triangle.
| ∆ABC | \angle A + \angle B + \angle C = 60\degree +60\degree + 60\degree =180\degree |
| ∆JKL | \angle J + \angle K + \angle L = 40\degree +90\degree + 50\degree =180\degree |
| ∆XYZ | \angle A + \angle B + \angle C = 40\degree +90\degree + 60\degree =180\degree |
| ∆MLN | \angle M + \angle L + \angle N = 50\degree +70\degree + 70\degree =190\degree |
From the table, we can see that the interior angles of the first three triangles all each have a sum of 180o. However, in ∆MLN, the sum of its interior angles is equal to 190o. This can’t be possible since the triangle sum theorem covers all triangles, so the interior angles of this triangle aren’t valid.
Before learning how to apply the triangle sum theorem and try out other problems, it’s important to understand how it’s possible that the interior angles of any triangle will always add up to 180o. Why don’t we take a look at its proof first?
Understanding the Triangle Sum Theorem’s Proof
Proving the triangle sum theorem begins by constructing a line that passes through one of the vertices. This line must also be parallel to the side of the triangle facing opposite the vertex that the line passes through. Use the properties of parallel lines to show that the sum of the three interior angles is 180o.

Now, observe the angles of this figure starting with the ones near the vertex, A. The two angles, \angle NAC and \angle CAB, form the larger angle, \angle NAB. Since MN is a line, the two angles that form it are linear pair of angles. Here are the relationships that have been established so far:
\begin{aligned}\angle NAB &= \angle NAC + \angle CAB\,\, (1)\\\angle NAB + \angle MAB &= 180\degree\,\,(2)\end{aligned}Use the right-hand side of the first equation to rewrite the expression for \angle NAB in the second equation.
\begin{aligned}(\angle NAC + \angle CAB) + \angle MAB &= 180\degree \end{aligned}Recall that alternate interior angles of parallel lines cut by a transversal line will have equal measures. Now, since MN and BC are parallel lines, we have the following pairs of interior angles: \angle MAB =\angle ABC and \angle NAC =\angle ACB. Use these relationships to rewrite the previous equation so that the right-hand side will have all the interior angles of ∆ABC.
\begin{aligned}\angle NAC + \angle CAB + \angle MAB &= 180\degree\\ \angle ACB +\angle CAB +\angle ABC &= 180\degree\end{aligned}Hence, it has been shown that the interior angles of a triangle will always have a sum of 180o. Now that the theorem has been proven for all triangles, it’s time to learn how to use this theorem to solve problems involving triangles and interior angles.
How To Solve Problems Using Triangle Sum Theorem?
When using the triangle sum theorem, identify the given interior angles and subtract the sum of these angles from 180o. Since triangles’ interior angles will always add up to 180o, the process of solving problems involving them will often require the triangle sum theorem.
Here are some steps to remember when solving problems using the triangle sum theorem:
Step 1: Identify the triangle’s three interior angles.
Step 2: Equate their sum’s expression to 180o.
Step 3: Solve the resulting equation to find the measure of the unknown interior angle.
Of course, the best way to master the triangle sum theorem is through practice. Work on the problems below to understand and appreciate this special property of interior angles within a triangle.
Problem 2
The two interior angles of a triangle have the following measures: 40o and 80o. What is the measure of the third interior angle?
The triangle sum theorem states that the sum of the three interior angles of the triangle is 180o, so the sum of 400, 80o, and the third angle (let it be x) must also be 180o.

Write the sum of the triangles in terms of x then equate the expression to 180o. This leads to an equation, so simplify it then solve for x.
\begin{aligned}40\degree + 80\degree + x\degree &= 180\degree\\ (120 + x)\degree &= 180\degree\\x &= 180 - 120\\x&= 60\end{aligned}This means that the third interior angle’s measure is 60o. Another way of approaching this problem is by working backward. We simply subtract the first angle from 180o then take out the second interior angle from the difference. This leaves us with the same measure for the third interior angle- 60o.

This approach is helpful when working quickly on numerical angle measures. If algebraic expressions are involved, stick with the first method for a more systematic approach to solving the problem.
Problem 3
Given the right triangle, ∆MNP, shown by the diagram below, what are the measures of \angle NMP and \angle MPN?

Right triangles will always have 90o as one of their interior angles. In fact, for ∆MNP, \angle MNP = 90\degree. This means that the sum of the two remaining interior angles is 90o. Add the two angles then equate it with 90o.
\begin{aligned}\angle MNP + \angle NMP + \angle MPN &= 180\degree\\90\degree + x\degree + (2x + 15)\degree &= 180\degree\\ x\degree + (2x + 15)\degree &= 90\degree\\3x + 15&= 90\\3x &= 75\\x&= 25 \end{aligned}This means that \angle NMP = x \degree = 25\degree. To find the measure of \angle PMN, substitute x =25 into the expression for the third interior angle.
\begin{aligned}\angle MPN &= \angle (2x + 15)\degree\\&= (2 \cdot 25 +15)\degree\\&= 65\degree\end{aligned}Hence, \angle MPN = 65\degree. To check if we got the right angle measures, add the three interior angles again and see if they add up to 180o.
\begin{aligned}\angle MNP + \angle NMP + \angle MPN &= 180\degree\\90\degree +25\degree +65 \degree&= 180\degree\\180\degree &\overset{\checkmark}{=} 180\degree \end{aligned}This confirms that the interior angles are correct. Apply a similar approach to solve more complex problems.
Problem 4
Some of the interior angles of the trapezoid, ABCD, are as shown below. If \angle BAD = \angle ADC, what is measure of \angle BDC?

As can be seen from the diagram, the trapezoid is composed of two triangles: ∆ADB and ∆BDC. Each set of interior angles for these two triangles will share the same sum: 180o. In ∆ADB, two of its interior angles’ measures are already given, so find the measure of the third angle. Subtract the measures of the two angles from 180o to find the measure of \angle ADB.
\begin{aligned}\angle ADB &= 180\degree - \angle DAB – \angle BAD\\&= 180\degree – 58\degree – 74\degree\\&= 48\degree\end{aligned}Now, we know that \angle BAD = \angle ADC, so \angle ADC = 58\degree. To find the measure of \angle BDC, subtract the measure of \angle ADB from that of \angle ADC.
\begin{aligned}\angle ADB + \angle DBC &= \angle ADC\\\angle ADB + \angle DBC&= 58\degree\\48 \degree + \angle BDC &= 58\degree\\\angle BDC &= 10\degree\end{aligned}Hence, \angle BDC has a measure of 10\degree.
