Hexagons are one of the most known two-dimensional figures. From the prefix, “hex”, which means six in Greek, hexagons are six-sided hexagons. It’s time that we learn how to identify and work with this important polygon – and see what makes hexagons unique!
There are a lot of objects in the real world that are hexagonal in shape. One of the reasons why we use hexagonal figures is that they stack up easily and form a tessellation. This is why knowing about their definition, properties, and formulas will come in handy when working with problems and applications involving hexagons.
This article covers its definition and properties thoroughly. We want to make sure that by the end of the discussion, you’ll feel confident when classifying hexagons, laying out their properties by heart, and when calculating hexagons’ perimeter and area.
What Are Hexagons?
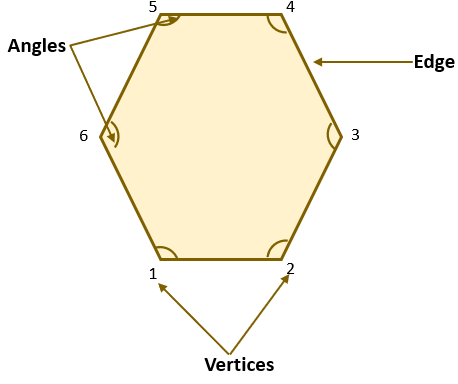
Hexagons are polygons enclosed by six sides. We’ve learned that polygons are two-dimensional figures enclosed by straight lines, so hexagons are 2D figures enclosed by six straight lines. Hexagons also have six vertices, edges, and angles each.
There are a lot of objects in our daily lives that are shaped like hexagons. Here are some examples of regularly-shaped hexagons that we encounter frequently.
- Stop signs are normally shaped as a circle, regular hexagon, or octagon.
- Honeycombs are one of the most common examples of hexagons.
- Snowflakes are also known for their hexagonal features. In fact, each snowflake will have six sides and six symmetrical folds.

Why is it that nature and man-made structures benefit from hexagonal figures? It has been shown that using hexagons can help maximize the area since it fills planes equally and compactly. With its shape, the perimeter is also minimized making it an ideal shape when stacking or packing items. Try to think of other objects that are hexagonal in shape – and you’ll be amazed yourself! This is why it’s helpful to know how to identify hexagons and understand their properties. We begin by learning how to classy hexagons into two types of classifications: based on their side lengths or their angle measures.
Two Types of Hexagons
We begin by learning how to classify hexagons into two types: as regular hexagons or as irregular hexagons. The images shown below highlight what makes one unique from the other.
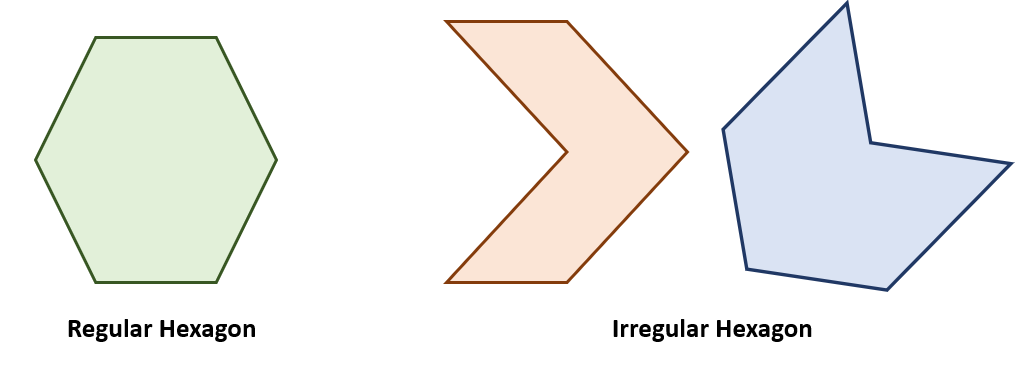
- Regular hexagons are hexagons that have six equal sides and six equal angles measuring 120\degree.
- Irregular hexagons are hexagons with varying measures for their sides and lengths.
Both regular and irregular hexagons’ angles will always add up to 720\degree while their external angles will always have a sum of 360\degree.
There is another way to classify hexagons based on their shapes – by classifying them as convex or concave hexagons. This time, we look at the hexagons’ angles and whether they’re pointing inwards or outwards.
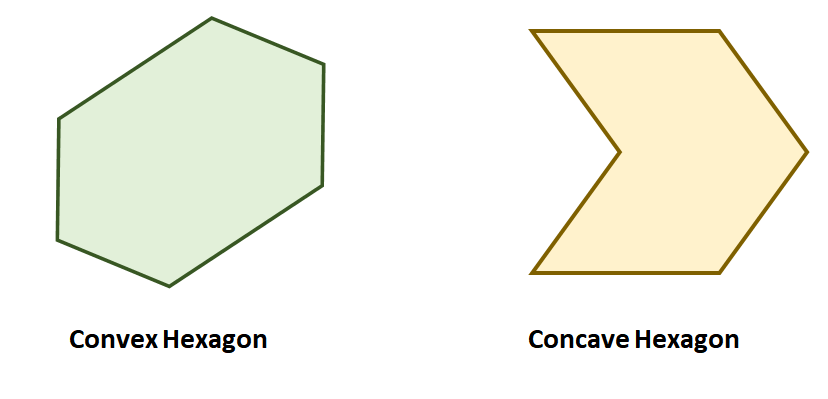
- Convex hexagons are hexagons where all interior angles are less than 180\degree. In addition, all the interior angles are pointing outwards.
- Concave hexagons, on the other hand, are hexagons that contain one or more interior angles that are greater than 180\degree. This means that some angles may point inwards.
These are the two most common ways to classify hexagons. Knowing these classifications will come in handy when working with applications and word problems involving hexagonal figures. In advanced geometry, identifying convex and concave hexagons will be important when proving theorems and solving for unknown values. For now, let’s test our understanding by trying out the problem below.
Problem 1
Determine whether the following statements are true or false.
a. From the figures shown below, Figure A is a hexagon.

First, ensure that these three figures are polygons by checking whether the sides fully enclose each figure. Now that we’ve confirmed that these are indeed polygons, let’s count the number of sides for each figure. Figure A has six sides, Figure B has five sides, and Figure C has four sides. From this alone, we know that only Figure A is a polygon, so the statement is true.
b. A concave hexagon may have an angle measure of 220\degree.
We’ve learned that concave hexagons will have angles each measuring less than 180\degree, so it is impossible for a concave hexagon to have an angle that measures 220\degree. This means that the statement is false.
c. The hexagon shown below is an irregular concave hexagon.

The hexagon shown above does have identical side lengths, so we can classify it as an irregular hexagon. Since one of its interior angles is pointing inwards, the hexagon is a convex hexagon. This means that the figure shown above is in fact an irregular convex hexagon making the statement is false.
We’ve covered all our bases when identifying regular and irregular hexagons as well as concave and convex hexagons. Let’s now focus on the most used and known type of hexagon: the regular hexagon. It’s time we understand what makes regular hexagons special and the interesting properties that they exhibit.
What Are the Properties of Regular Hexagons?
Regular hexagons, as we have learned, will always have equal side lengths and equal angle interior angles. They also exhibit a lot of interesting properties and this section will cover the essential ones we need to know about regular hexagons.
- The sum of the interior angles of any hexagon will always be 720\degree, so the interior angles of a regular hexagon will each measure 720\degree \div 6 = 120\degree.

- Regular hexagons also exhibit six lines of symmetry and they can be rotated six times before they go back to their original positions.

Aside from these properties, it’s important that we know how to find the perimeter and area of regular hexagons. Let’s use the regular hexagon’s definition and properties to establish the respective formulas for the hexagon’s perimeter and area.
Areas and Perimeters of Regular Hexagons
In general, we simply add the side lengths of hexagons to find their perimeter. Since a regular hexagon has six equal sides, we can simply multiply one of its sides by six to find its perimeter.
\begin{aligned}\textbf{Perimeter} &= 6s \end{aligned}Now, let’s establish the rules for finding the regular hexagon’s area given its side. Since regular hexagons can be divided into six equilateral triangles, we can use the special triangle, 30\degree– 60\degree– 90\degree, to find the height of one triangle.

This means that one equilateral triangle has an area of \frac{\sqrt{3}}{4}s^2. The regular hexagon’s area will simply be equal to six times the area of one triangle.
\begin{aligned}\textbf{Area} &= 6 \times \dfrac{\sqrt{3}}{4}s^2\\&=\dfrac{3\sqrt{3}}{2}s^2 \end{aligned}We call the height of the triangle as the regular hexagon’s apothem. When we’re given the hexagon’s apothem,a, we can easily find the hexagon’s area using its apothem and perimeter.
\begin{aligned}\textbf{Area} &= \dfrac{1}{2}aP \end{aligned}For this formula, P represents the hexagon’s perimeter. We’ve established the rules and properties involving regular hexagons, so it’s time for us to check our knowledge by answering the problems shown below.
Problem 2
Suppose that a regular hexagon has a side length of 12 cm. What is the regular hexagon’s perimeter and area?
Since we’re working with a regular hexagon, all its six sides will share the same length. To find its perimeter, we simply need to multiply the side length by six.
\begin{aligned}\textbf{Perimeter} &= 6 \times 12\\&= 72\end{aligned}This means that the regular hexagon has a perimeter of 72 cm. Now to find its area, we simply apply the formula and use s = 6 cm.
\begin{aligned}\textbf{Area} &= \dfrac{3\sqrt{3}}{2}s^2\\&= \dfrac{3\sqrt{3}}{2}(6)^2\\&= 54\sqrt{3} \end{aligned}The regular hexagon has an area of 54\sqrt{3} \approx 93.53 cm2.
Problem 3
A regular hexagon has an area of 72\sqrt{3} ft2. What is its perimeter?
Recall that the formulas for the hexagon’s perimeter and area are all dependent on its side length. For this problem, we’ll need to work backwards to find the length of one side of the regular hexagon.
\begin{aligned}\textbf{Area} &= \dfrac{3\sqrt{3}}{2}s^2\end{aligned}Use \textbf{Area} = 72\sqrt{3} ft2 and find the value of s or the hexagon’s side length.
\begin{aligned}\text{Area}&=\dfrac{3\sqrt{3}}{2}s^2\\72\sqrt{3}&=\dfrac{3\sqrt{3}}{2}s^2\\72\sqrt{3} \cdot \dfrac{2}{4\sqrt{3}}&= s^2\\s^2 &= 36\\s&= 6 \end{aligned}From this, we can see that the regular hexagon has a side length of 6 ft. To find the regular hexagon’s perimeter, we simply multiply the side length by 6.
\begin{aligned}\text{Perimeter} &= 6s\\&= 6(6)\\&= 36 \end{aligned}This means that the regular hexagon has a perimeter of 36 ft2.
Problem 4
What is the area of the hexagon given that it has a side length of 12 m and an apothem measuring 6\sqrt{3} m?
We can find the area of a hexagon using its apothem and perimeter, so we begin by calculating its perimeter.
\begin{aligned}\textbf{Perimeter} &= 6 \times 12\\&= 72\end{aligned}Now that we have its perimeter, we simply multiply the regular hexagon’s apothem to 72 m2.
\begin{aligned}\text{Area} &= \dfrac{1}{2}aP\\&= \dfrac{1}{2}(6\sqrt{3})(72)\\&= 216\sqrt{3}\\&\approx 374.12 \end{aligned}This means that the regular hexagon has an area of 216\sqrt{3} m2 or approximately 374.12 m2.
These three problems show you how to use the different formulas when calculating a regular hexagon’s area and perimeter. Keep your notes on hexagons and we’ll guarantee that these will come in handy later!
